
The result is valid for both a horizontal and a vertical axis through the centroid, and therefore is also valid for an axis with arbitrary direction that passes through the origin.
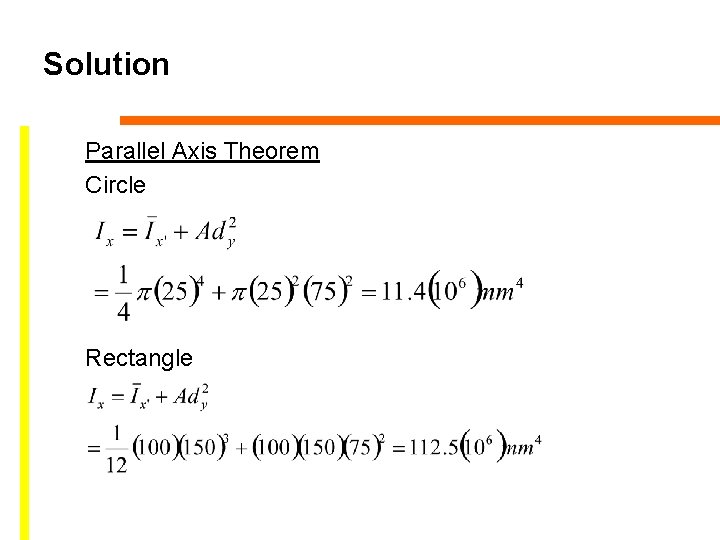
If is a point in the plane of an area and distant from the centroid of the area as shown in Fig. Apply the parallel axis theorem to find the moment of inertia about any axis parallel to one already known Calculate the moment of inertia for compound objects. The parallel axis theorem also hold for the polar moment of inertia. Moment of Inertia: Cylinder About Perpendicular Axis The development of the expression for the moment of inertia of a cylinder about a diameter at its end (the x-axis in the diagram) makes use of both the parallel axis theorem and the perpendicular axis theorem. Equations C-11 are called parallel-axis theorem for moments of inertia. Where is the distance between the two parallel axes. For simple geometric shapes (e.g., rectangles, triangles, circles) there are. The so-called Parallel Axes Theorem is given by the following equation: where I' is the moment of inertia in respect to an arbitrary axis, I the moment of inertia in respect to a centroidal axis, parallel to the first one, d the distance between the two parallel axes and A the area of the shape. If is an axis crossing, and a parallel axis to as shown in Fig. Įquation 10.7 can be written for any two parallel axes with one crossing the centroid of the area. The moment of inertia, otherwise known as the mass moment of inertia, angular mass, second moment of mass, or most accurately, rotational inertia, of a rigid body is a quantity that determines the torque needed for a desired angular acceleration about a rotational axis, akin to how mass determines the force needed for a desired acceleration.
#Moment of inertia of a circle parallel axis theorem plus#
Which reads the moment of inertia about an axis is equal to the moment of inertia about a parallel axis that crosses the centroid of, plus the product of area and the square distance between and. The term equals zero because and (measured from the axis) because passes through the centroid. It states, If Ivy and ly be the moments of inertia of a plane section. If is a differential element of the area, its (perpendicular) distance to the axis can be written as where is the distance between the two parallel axes shown in Fig. Am bout two perpendicula unicular to the plane and. 10.9 Terms involved in deriving the parallel axis theorem. Derivation of mass moment of inertia for. According to the parallel axis theorem 4 4 V. where, I is the moment of inertia about the arbitrary axis, I CM is the moment of inertia about the parallel axis through the centre of mass, M is the total mass of the object, r is the perpendicular distance between the axis.
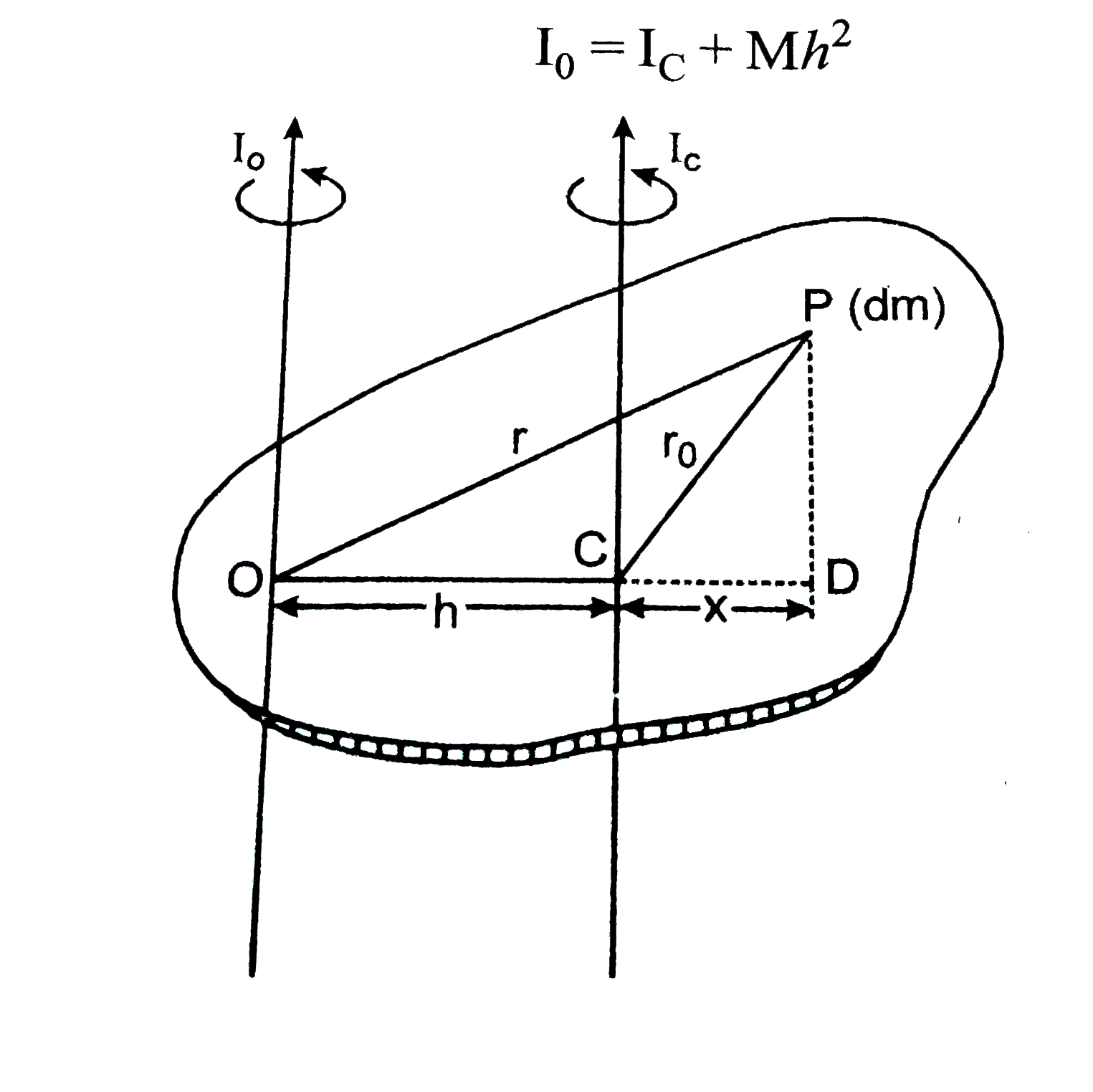
Relationships between Load, Shear, and Momentsįig. Parallel axis theorem and perpendicular axis theorem Polar moment of inertia Mass moment of inertia. Parallel axis Theorem Diagram: Mathematically, I I CM + Mr.Shear and moment equations and their diagrams.Conditions for two dimensional rigid-body equilibrium Area Moments of Inertia Parallel Axis Theorem Consider moment of inertia I of an area Awith respect to the axis AA’I y2dA The axis BB’ passes through the area centroid and is called a centroidal axis.Equilibrium of Particles and Rigid Bodies.Simplification of force and couple systems.Vector operations using Cartesian vector notation.

For a material point of mass m, which rotates around an axis at a distance r, this formula will have the following form: Huygens-Steiner Theorem Speaking about the moment of inertia, it is impossible.



 0 kommentar(er)
0 kommentar(er)
